FIGURAS CÓNICAS

Mer av detta

Asiento Contable

av Diana Peña

la hipérbola

av Karla Estrada

FUNCIONES REALES DE VARIABLE REAL

av Betuel Castillo

Ley de la conservacion de la materia

av SOLANO PEREZ ANGEL GABRIEL

FIGURAS CÓNICAS

Type in the name of the multiple-perspectives text.

Example: Bridge to Terabithia by Katherine Paterson

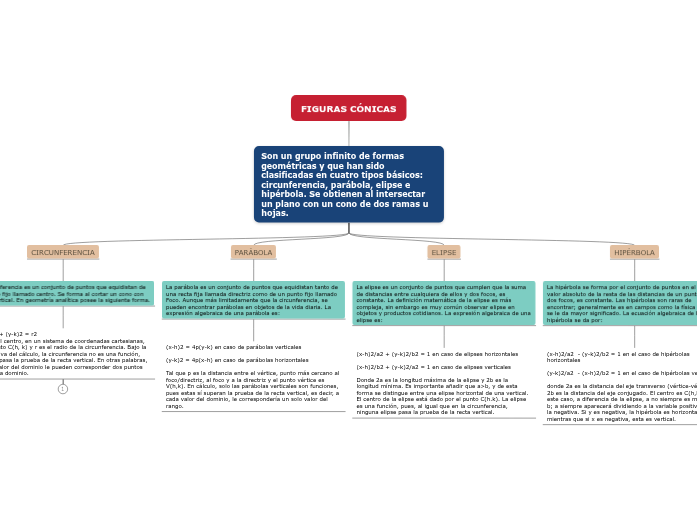
Son un grupo infinito de formas geométricas y que han sido clasificadas en cuatro tipos básicos: circunferencia, parábola, elipse e hipérbola. Se obtienen al intersectar un plano con un cono de dos ramas u hojas.

Identify an important issue from the text that is being presented from different angles. Type it in.

Example: Jesse's drawing talent.

HIPÉRBOLA

Decide on the fourth point of view

Type in the name of the last character whose perspective on the issue you are going to present.

Example: Leslie Burke, Jesse's new next-door neighbor, and best friend.

La hipérbola se forma por el conjunto de puntos en el que el valor absoluto de la resta de las distancias de un punto con dos focos, es constante. Las hipérbolas son raras de encontrar; generalmente es en campos como la física donde se le da mayor significado. La ecuación algebraica de la hipérbola se da por:

Point of view

Type in a relevant quote that highlights the character's point of view. Try to follow a citation format: author's name, chapter, and page.

Example: I can't get the poetry of the trees,' he said. She nodded. Don't worry,' she said. You will someday. He believed her.' (Paterson, 4. 24)

(x-h)2/a2 - (y-k)2/b2 = 1 en el caso de hipérbolas horizontales (y-k)2/a2 - (x-h)2/b2 = 1 en el caso de hipérbolas verticales donde 2a es la distancia del eje transverso (vértice-vértice) y 2b es la distancia del eje conjugado. El centro es C(h,k). En este caso, a diferencia de la elipse, a no siempre es mayor que b; a siempre aparecerá dividiendo a la variable positiva y b a la negativa. Si y es negativa, la hipérbola es horizontal, mientras que si x es negativa, esta es vertical.

ELIPSE

Whose character does the third point of view belong to?

Type in his/her name.

Example: Mr. Aarons, Jesse's father.

La elipse es un conjunto de puntos que cumplen que la suma de distancias entre cualquiera de ellos y dos focos, es constante. La definición matemática de la elipse es más compleja, sin embargo es muy común observar elipse en objetos y productos cotidianos. La expresión algebraica de una elipse es:

What does the character think, say or do that suggests their perspective on the issue?

Type in a quote and try to maintain the citation format.

Example: 'He would like to show his drawings to his dad, but he didn't dare. (...) He'd thought his dad would be pleased. He wasn't. What are they teaching in that damn school? he had asked.' (Paterson, 2.8)

(x-h)2/a2 + (y-k)2/b2 = 1 en caso de elipses horizontales (x-h)2/b2 + (y-k)2/a2 = 1 en caso de elipses verticales Donde 2a es la longitud máxima de la elipse y 2b es la longitud mínima. Es importante añadir que a>b, y de esta forma se distingue entre una elipse horizontal de una vertical. El centro de la elipse está dado por el punto C(h.k). La elipse es una función, pues, al igual que en la circunferencia, ninguna elipse pasa la prueba de la recta vertical.

PARÁBOLA

Decide on the second point of view

Name the character (it can either be the main character or one of the supporting characters) whose point of view you are presenting.

Example: Miss Edmunds, Jesse's music teacher.

La parábola es un conjunto de puntos que equidistan tanto de una recta fija llamada directriz como de un punto fijo llamado Foco. Aunque más limitadamente que la circunferencia, se pueden encontrar parábolas en objetos de la vida diaria. La expresión algebraica de una parábola es:

Type in a quote that points out the character's position about the issue.

Try to follow a citation format: author's name, chapter, and page.

Example: 'She said he was unusually talented, and she hoped he wouldn't let anything discourage him.' (Paterson, 2. 8)

(x-h)2 = 4p(y-k) en caso de parábolas verticales (y-k)2 = 4p(x-h) en caso de parábolas horizontales Tal que p es la distancia entre el vértice, punto más cercano al foco/directriz, al foco y a la directriz y el punto vértice es V(h,k). En cálculo, solo las parábolas verticales son funciones, pues estas sí superan la prueba de la recta vertical, es decir, a cada valor del dominio, le correspondería un solo valor del rango.

CIRCUNFERENCIA

Decide on the first point of view you are going to present.

Type in the name of the character (it can either be the main character or one of the supporting characters) whose point of view belongs to.

Example: Jesse Oliver Aarons, Jr., the main character of the novel, a fifth-grader living in a rural Southern area.

La circunferencia es un conjunto de puntos que equidistan de un punto fijo llamado centro. Se forma al cortar un cono con plano vertical. En geometría analítica posee la siguiente forma.

Type in a relevant quote that highlights the character's point of view towards
Son un grupo infinito de formas geométricas y que han sido clasificadas en cuatro tipos básicos: circunferencia, parábola, elipse e hipérbola. Se obtienen al intersectar un plano con un cono de dos ramas u hojas..

Try following a citation format: author's name, chapter, and page.

Example: 'Jesse drew the way some people drank whiskey. (...) Lord, he loved to draw. (...) When he was in first grade, he told his father that he wanted to be an artist when he grew up.' (Paterson, 2. 7)

(x - h)2 + (y-k)2 = r2 Tal que el centro, en un sistema de coordenadas cartesianas, es el punto C(h, k) y r es el radio de la circunferencia. Bajo la perspectiva del cálculo, la circunferencia no es una función, pues no pasa la prueba de la recta vertical. En otras palabras, a cada valor del dominio le pueden corresponder dos puntos del contra dominio.