Operation of Division
Measurement Model
(Repeat subtraction)
characterized by using a given quantity to creat groups (partitions) of a specified size (amount) and determining the number of partitions (groups) that are formed.
Know:
Quantity we are starting with
Size of each group
Find:
The number of groups
Characterized by distributing a given quantity amoung a specified number of groups (partitions) and determining the size (or amount) in each group ( partition)
Know:
Quantity we are starting with
The number of groups
Find:
the size of each group (how many)
Peugeot Proportional Reasoning
Ratio Sense
Relative thinking
Quantities
Unitizing
Properties of Subtraction
Identity Prop of Subtraction
If a ∈ W then a - 0 = a = 0 - a
let a = 1 then 1 - 0 = 1 but 0 - 1 += -1
a - 0 = a 0 - a ≠ a
Communitive Prop of Subtraction
If a ∈ W and b ∈ W, then (a-b) = (b - a)
Let a = 1, b = 3 then a - b = -2 b - a = 2
a - b ≠ b - a
Closure Property of Subtraction
If a ∈W and b ∈ W, then (a-b) ∈ W
Let a = 1, b = 3 then 1 - 3 ∈ W
Mindomo 4/13 through 4/26
Properties of Modular Arthrimitic
Inverse Property
a+ b (m5) = 0
Identity Property
a + ____ (m5) = a
Communitive Property
Move or transpose
3+4 = 4+3
Mirror images
Closure Prop of addition
3(five) + 4 (five) = 12 (five)
3(m5) + 4 (m5) = 2 (only single digits 0,1,2,3,4)
Modular Clock
Equivalent (three bars) or congruent
Look at remainder, not the divisor
(scant notes/substitute)
Properties of Whole Number Operations
Distributive Prop of Multiplication over add/sub
a ∈ W, b ∈ W, c ∈ W, then a * (b+c) = ab + ac
Zero Property of Multiplication
The effect of multiplying by zero
a ∈ W, then 0 * a = 0
One of the numbersmust be zero
Identity Property of Mult.
multiply by the same number, you get the identical thing (by 1)
a ∈ W, then 1 * a = a
1 is the identity element or multiplicative element
Associative Prop of Multiplication
a ∈ W, b ∈ W, c ∈ W, then (a*b) * c - a * (b * c)
(changes the group)
Communitive Prop of Multiplication
a ∈ W, b ∈ W then ab = ba
(changes the order)
Closure Property for Multiplication
a ∈W, b ∈ W then a * b ∈ W
Operation of Multiplcation
Carteisan Product
Context is characterized by finding all possible pairings between 2 or more sets of objects
(cross product to come up with ordered pairs)
Area Model/Array
characterized by a product of two numbers representing the sides of a rectangular region such that the product represents the number of unit sized squares within the rectangular region.
Repeated addition continuous
characterized by repeatedly adding a quantity of continuous quantities. Measured quantity like time, distance, etc. a specified number of times.
Mult as repeated addition
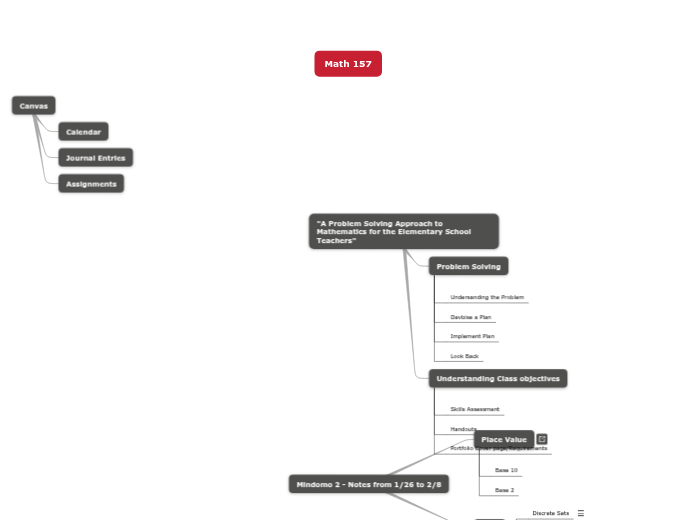
Canvas
Assignments
Journal Entries
Calendar
Mindomo 5 - notes from 3/30 through 4/12
Proportional Reasoning
Proportion
Rate
Ratio
Models of division
Repeated Subtraction
Know: Size of groups
Find: number of groups
Partition Model
Know: Number of groups/partition
Find: size of partition
Area model of multiplication
How to work fraction problems
converting mixed numbers:
ADV: no regrouping of fractions needed.
Looks like part-whole context
Process is similar to mult/div.
Dis: Larger numbers, more opportunity for math mistakes
Specficially when converting between 2 forms.
Use mult/div within problem.
leaving mixed numbers
ADV: no converstion, less work.
Strengthens the idea of place value
More consistent
Dis; Regrouping of fractions, particularly - what is the whole?
Mindomo 3 - Notes from 2/9 through 2/22
Traditional Algorithm Subtraction
Addition Tables
Structure/Patterns in Addition Tables
Diagonally numbers appear in bands. All possible ways add 2 numbers and get 10.
Traditional Algorithm Addition
The traditional algorithm is efficient and saves space. It is suited to the resources available.
Lattice Method
Scratch Method
Regrouping
Properties of Addition
Identity Property Of Addition
If a ∈W, then a+ 0 = a = 0 + a
The identity element for addition is zero.
Associative Prop of Addition
coming together, pair up
if a € W, b € W, and c € W, then
(a+b) + c = A+ (b+c) = (a+c) +b
Communitive Prop of Addition
If a € W and b € W then a+b = b+a
Closure Property of Addition
If a is an element of set x and b is an element of set x, then a plus b is an element of set x
Of whole numbers:
if a € W and b € W then a+b € W
Mindomo 4 - notes from 3/19 through 3/29
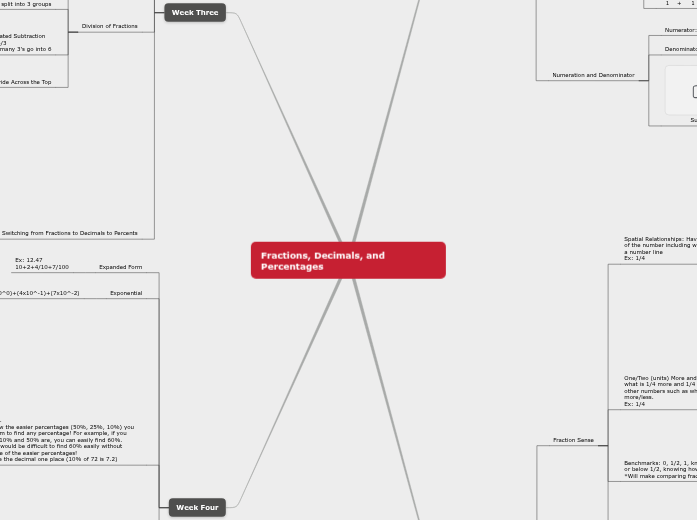
Fractions
Identifying the whole
Be careful of when you need to think about a different "whole" (yep, I still call it switching the whole.)
Realtive size of Fractions
Rational numbers
Any number that can be expressed as the quotient of 2 integers. Includes repeating decimals etc
Mutlple Intepretation of Fractions
Part to whole meaning - most common
Division - in building math sophistication we remove the symbol. The fraction bar eventually becomes an alternative too for indicating division
Copies of a unit fraction (supposed to accompany part/whole.
Ration - involves comparing 2 separate things.
"A Problem Solving Approach to Mathematics for the Elementary School Teachers"
Understanding Class objectives
Portfolio Cover page/Requirements
Handouts
Skills Assessment
Problem Solving
Look Back
Implement Plan
Devbise a Plan
Undersanding the Problem
Mindomo 2 - Notes from 1/26 to 2/8
Sets
Continuous Sets
Continuous sets - characterized by combining of 2 continous quantities, flowing quantities that we measure. i.e. time, distance, volume, area
Discrete Sets
Discrete Sets - characterized by combining 2 sets of counted quantities i.e. blocks, markers.
Place Value
Base 2
Base 10
Mindomo 4 - Noted from 2/23 through 3/08
Cuisenaire Rods
Least Common Multiple
Greatest Common Factor
Number Theory
Odd
An odd number is one more/less than an even number.
That is, 2n+1 (2n-1 is also valid)
Even
What make a number even?
A number is even if it is a multiple of 2. That is, it is 2 times some number or 2n.
Four Equivalent Statements
Assuming b divides a, then:
- b is a factor of a
- a is a multiple of b
- b is a divisor of a
- a is divisible by b.
Divisibility
Definition: A whole number a is divisible by a whole number b, if and only if, there exists a third whole number c such that a = bc.
Often stated as b divides a and the notation is bIa
Scaffolding Method
Column Division
Partial - Quotients Method
Array Division
Math 157