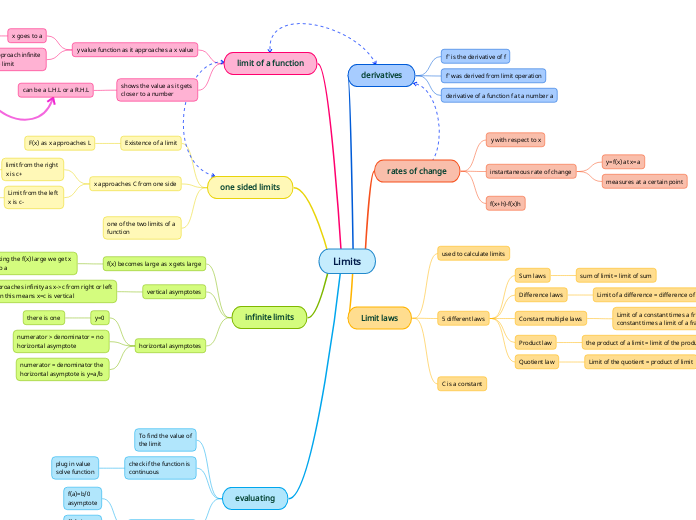
f' is the derivative of f
f' was derived from limit operation
derivative of a function f at a number a
y with respect to x
instantaneous rate of change
y=f(x) at x=a
measures at a certain point
f(x+h)-f(x)h
used to calculate limits
5 different laws
Sum laws
sum of limit = limit of sum
Difference laws
Limit of a difference = difference of a limit
Constant multiple laws
Limit of a constant times a fraction =
constant times a limit of a fraction
Product law
the product of a limit = limit of the product
Quotient law
Limit of the quotient = product of limit
C is a constant
y value function as it approaches a x value
x goes to a
x is variable and a is constant
If both sides approach infinite
its to be infinite limit
shows the value as it gets
closer to a number
can be a L.H.L or a R.H.L
Existence of a limit
F(x) as x approaches L
x approaches C from one side
limit from the right
x is c+
Right hand limit
the limit is greater then
x=a+h where h -> 0
Limit from the left
x is c-
left hand limit
the value is less then
x=a-h where h>0 such
h-> 0
one of the two limits of a
function
f(x) becomes large as x gets large
By making the f(x) large we get x
close to a
vertical asymptotes
approaches infinity as x->c from right or left
then this means x=c is vertical
factor does not show in the
numerator its vertical
horizontal asymptotes
y=0
there is one
numerator > denominator = no
horizontal asymptote
numerator = denominator the
horizontal asymptote is y=a/b
To find the value of
the limit
check if the function is
continuous
plug in value
solve function
Try to evaluate a limit
f(a)=b/0
asymptote
f(a)=b
limit found
F(a)=0/0
indeterminate form
With this rewrite limit